问题
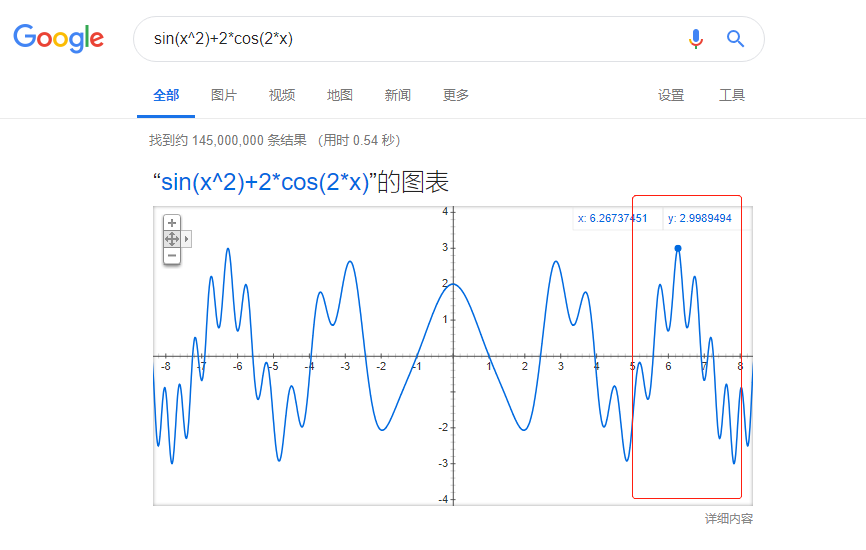
找图中函数在区间[5,8]的最大值
重点思路
爬山算法会收敛到局部最优,解决办法是初始值在定义域上随机取乱数100次,总不可能100次都那么倒霉。
实现
|
1
2
3
4
5
6
7
8
9
10
11
12
13
14
15
16
17
18
19
20
21
22
23
24
25
26
27
28
29
|
import numpy as npimport matplotlib.pyplot as pltimport math# 搜索步长delta = 0.01# 定义域x从5到8闭区间bound = [5,8]# 随机取乱数100次generation = 100def f(x): return math.sin(x*x)+2.0*math.cos(2.0*x)def hillclimbing(x): while f(x+delta)>f(x) and x+delta<=bound[1] and x+delta>=bound[0]: x = x+delta while f(x-delta)>f(x) and x-delta<=bound[1] and x-delta>=bound[0]: x = x-delta return x,f(x)def findmax(): highest = [0,-1000] for i in range(generation): x = np.random.rand()*(bound[1]-bound[0])+bound[0] currentvalue = hillclimbing(x) print('current value is :',currentvalue) if currentvalue[1] > highest[1]: highest[:] = currentvalue return highest[x,y] = findmax()print('highest point is x :{},y:{}'.format(x,y)) |
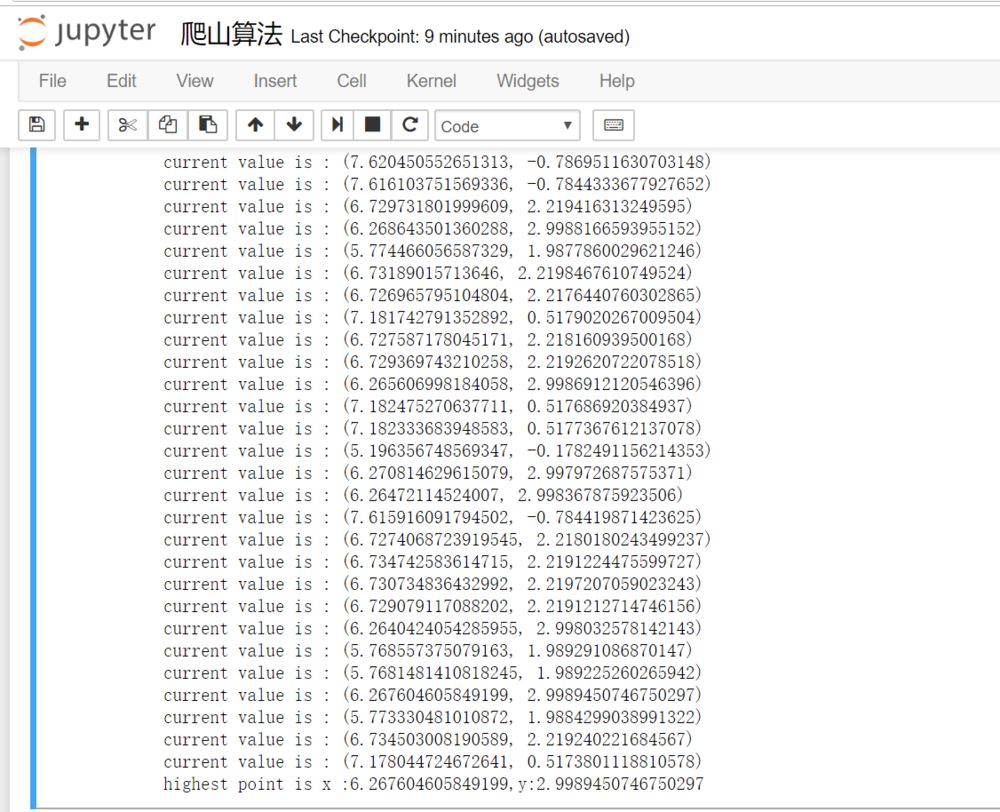
运行结果:
总结
以上所述是小编给大家介绍的python实现爬山算法的思路详解,希望对大家有所帮助,如果大家有任何疑问欢迎给我留言,小编会及时回复大家的!
原文链接:https://www.jianshu.com/p/70b4c878917e